Math word problems are essential tools for developing critical thinking skills in students. They help bridge the gap between theoretical concepts and real-world applications, enabling learners to grasp how math plays a role in everyday life. From basic addition and subtraction to more complex topics like ratios and proportions, these problems encourage students to think logically and creatively.
In this blog, you’ll find a comprehensive collection of math word problems categorized by grade levels, from kindergarten to Grade 8. Each section provides a variety of problems that challenge students at their respective levels, offering valuable practice to enhance their mathematical skills.
But first, let’s break down a quick guide on dos and don’ts in tackling math word problems for effective teaching.
- Do read the problem carefully to students. Ensure students fully understand what is being asked.
- Do encourage students to identify and highlight key information, such as important numbers and operational keywords.
- Don’t rush through the process. Remind students that taking time to think through each step leads to more accurate solutions.
- Don’t overlook the importance of units. Emphasizing units of measurement helps students avoid calculation errors.
- Do integrate a variety of problems into your lessons regularly. This approach builds students’ confidence and helps them become more adept at problem-solving.
Kindergarten to Grade 2 Math Word Problems

Basic Addition and Subtraction
❓ Problem 1:
Lily has 3 apples, and she picks 5 more from the tree. How many apples does she have now?
✅ Solution: 3 + 5 = 8 apples
❓ Problem 2:
John had 7 candies, but he ate 4. How many candies does he have left?
✅ Solution: 7 – 4 = 3 candies
❓ Problem 3:
There are 6 ducks in a pond, and 3 more ducks join them. How many ducks are there in total?
✅ Solution: 6 + 3 = 9 ducks
❓ Problem 4:
Sarah had 8 crayons, but she gave 2 to her friend. How many crayons does Sarah have now?
✅ Solution: 8 – 2 = 6 crayons
❓ Problem 5:
A pet shop has 4 cats and 7 dogs. How many pets are there altogether?
✅ Solution: 4 + 7 = 11 pets
❓ Problem 6:
Tommy had 9 balloons, but 5 of them flew away. How many balloons does Tommy have now?
✅ Solution: 9 – 5 = 4 balloons
❓ Problem 7:
There are 12 birds sitting on a tree. 3 birds fly away. How many birds are left on the tree?
✅ Solution: 12 – 3 = 9 birds
❓ Problem 8:
Mary buys 6 cupcakes, and her friend gives her 3 more. How many cupcakes does Mary have now?
✅ Solution: 6 + 3 = 9 cupcakes
❓ Problem 9:
Alex has 10 marbles, and he gives 4 to his friend. How many marbles does Alex have left?
✅ Solution: 10 – 4 = 6 marbles
❓ Problem 10:
In a garden, there are 7 red flowers and 2 yellow flowers. How many flowers are there in total?
✅ Solution: 7 + 2 = 9 flowers
💡 Bonus Interactive Tip
Cut the traditional pen and paper. If you’re actively using PowerPoint to carry out lessons, use ClassPoint to engage students in solving math word problems.
Take advantage of ClassPoint’s interactive quiz question type, Short Answer, where you can send problem slides directly from your PowerPoint slides to your students’ devices. They can respond in real-time, submitting their answers that automatically appear back on your presentation.
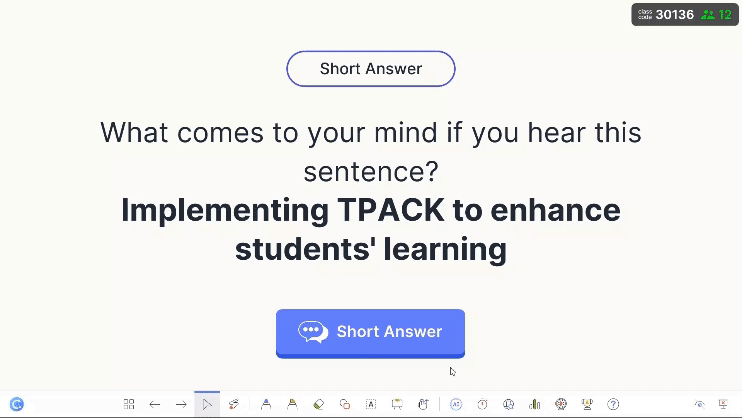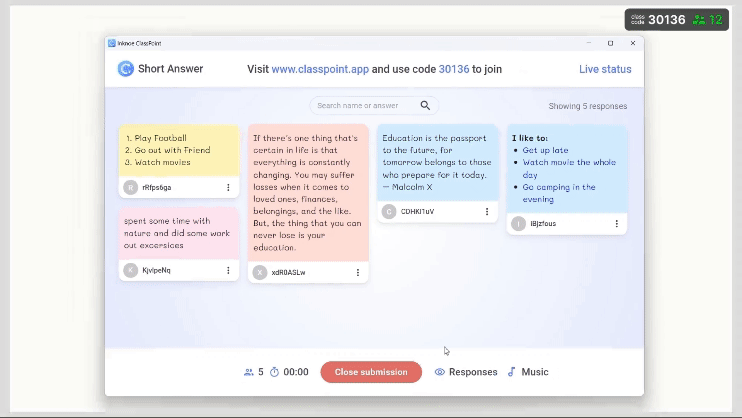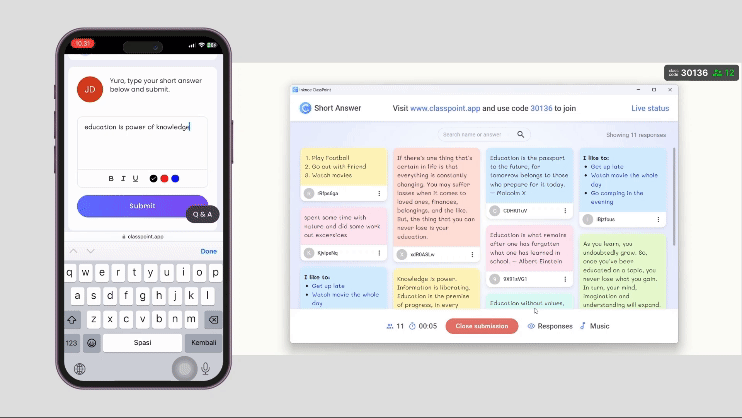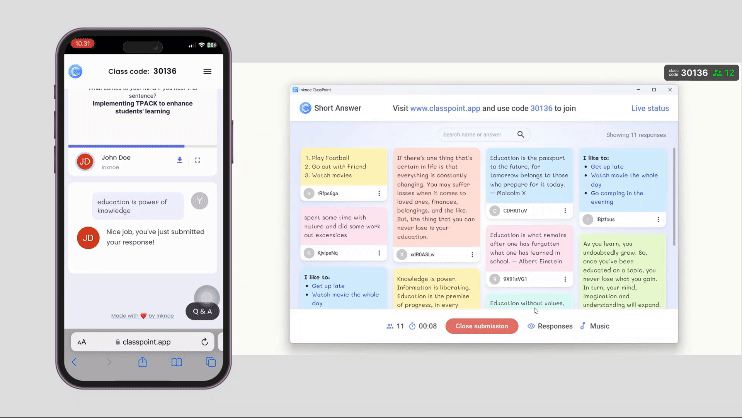
Recognize correct answers by awarding stars, which students can accumulate to unlock levels and earn badges—adding a gamified touch.
At the end of the session, display a visual leaderboard directly in PowerPoint, showcasing student achievements and encouraging a healthy sense of competition.

Simple Multiplication Math Word Problems
❓ Problem 11:
There are 2 baskets, and each basket has 3 oranges. How many oranges are there in total?
✅ Solution: 2 × 3 = 6 oranges
❓ Problem 12:
Sam has 4 boxes of toys. Each box contains 2 toys. How many toys does Sam have?
✅ Solution: 4 × 2 = 8 toys
❓ Problem 13:
There are 3 shelves, and each shelf has 5 books. How many books are there in total?
✅ Solution: 3 × 5 = 15 books
❓ Problem 14:
Anna sees 5 cars, and each car has 4 wheels. How many wheels are there in total?
✅ Solution: 5 × 4 = 20 wheels
❓ Problem 15:
There are 2 students, and each student brings 6 pencils. How many pencils are there in total?
✅ Solution: 2 × 6 = 12 pencils
Counting and Numbers
❓ Problem 16:
What number comes after 7?
✅ Answer: 8
❓ Problem 17:
If you have 3 apples and someone gives you 2 more, how many apples do you have in total?
✅ Answer: 3 + 2 = 5 apples
❓ Problem 18:
Which number is greater: 5 or 3?
✅ Answer: 5
❓ Problem 19:
How many legs does a dog have?
✅ Answer: 4 legs
❓ Problem 20:
What number is between 2 and 4?
✅ Answer: 3
Basic Time and Measurement
❓ Problem 21:
If the clock shows 3 o’clock, how many hours will it be until 5 o’clock?
✅ Answer: 2 hours
❓ Problem 22:
You have a piece of string that is 12 centimeters long. If you cut it in half, how long is each piece?
✅ Answer: 6 centimeters
❓ Problem 23:
If it is 8:00 AM now, what time will it be in 2 hours?
✅ Answer: 10:00 AM
❓ Problem 24:
If it takes 30 minutes to walk to the park and you leave at 3:00 PM, what time will you arrive?
✅ Answer: 3:30 PM
❓ Problem 25:
How many minutes are there in an hour?
✅ Answer: 60 minutes
Simple Money Math Word Problems
❓ Problem 26:
Sara has 3 coins, and each coin is worth 1 peso. How much money does Sara have?
✅ Solution: 3 × 1 = 3 pesos
❓ Problem 27:
Tom buys a pencil for 5 pesos and an eraser for 3 pesos. How much money does he spend in total?
✅ Solution: 5 + 3 = 8 pesos
❓ Problem 28:
You have 10 pesos and you buy candy for 6 pesos. How much money do you have left?
✅ Solution: 10 – 6 = 4 pesos
❓ Problem 29:
If each apple costs 2 pesos, how much will 4 apples cost?
✅ Solution: 2 × 4 = 8 pesos
❓ Problem 30:
Ella has 7 pesos and buys a toy for 3 pesos. How much money does she have left?
✅ Solution: 7 – 3 = 4 pesos
Grades 3 to 5 Math Word Problems

Multiplication and Division
❓ Problem 31:
There are 4 boxes, and each box contains 8 chocolates. How many chocolates are there in total?
✅ Solution: 4 × 8 = 32 chocolates
❓ Problem 32:
A team has 24 players, and the coach divides them into groups of 6. How many groups are formed?
✅ Solution: 24 ÷ 6 = 4 groups
❓ Problem 33:
There are 9 students, and each student has 5 pencils. How many pencils are there in total?
✅ Solution: 9 × 5 = 45 pencils
❓ Problem 34:
Sam has 36 marbles. He divides them equally among 4 friends. How many marbles does each friend get?
✅ Solution: 36 ÷ 4 = 9 marbles
❓ Problem 35:
A bakery bakes 7 trays of cookies. Each tray holds 6 cookies. How many cookies are baked in total?
✅ Solution: 7 × 6 = 42 cookies
❓ Problem 36:
Emma has 28 apples, and she packs them into bags of 7. How many bags does she use?
✅ Solution: 28 ÷ 7 = 4 bags
❓ Problem 37:
If a garden has 5 rows of 9 flowers each, how many flowers are there in the garden?
✅ Solution: 5 × 9 = 45 flowers
❓ Problem 38:
A teacher has 40 markers. She divides them equally among 8 students. How many markers does each student get?
✅ Solution: 40 ÷ 8 = 5 markers
❓ Problem 39:
There are 6 baskets, and each basket has 7 oranges. How many oranges are there in total?
✅ Solution: 6 × 7 = 42 oranges
❓ Problem 40:
Lisa has 48 candies and divides them among her 6 friends equally. How many candies does each friend get?
✅ Solution: 48 ÷ 6 = 8 candies
Fractions and Decimals
❓ Problem 41:
What is 1/2 of 8?
✅ Solution: 8 ÷ 2 = 4
❓ Problem 42:
If you cut a pizza into 4 equal slices and eat 1 slice, what fraction of the pizza is left?
✅ Solution: 3/4 of the pizza is left
❓ Problem 43:
What is 1/4 of 20?
✅ Solution: 20 ÷ 4 = 5
❓ Problem 44:
If you have $1.50 and spend $0.75, how much money do you have left?
✅ Solution: $1.50 – $0.75 = $0.75
❓ Problem 45:
Which is greater: 0.8 or 0.5?
✅ Solution: 0.8 is greater
❓ Problem 46:
Sarah eats 3/8 of a cake. What fraction of the cake is left?
✅ Solution: 5/8 of the cake is left
❓ Problem 47:
If you drink 1/3 of a glass of milk, how much of the glass is left?
✅ Solution: 2/3 of the glass is left
❓ Problem 48:
Convert 0.25 to a fraction.
✅ Solution: 1/4
❓ Problem 49:
What is 2/3 of 12?
✅ Solution: 12 × 2/3 = 8
❓ Problem 50:
Add 0.6 and 0.4.
✅ Solution: 0.6 + 0.4 = 1
Geometry and Shapes
❓ Problem 51:
What is the sum of the interior angles of a quadrilateral?
✅ Answer: 360°
❓ Problem 52:
If a triangle has angles of 50° and 60°, what is the measure of the third angle?
✅ Answer: 70°
❓ Problem 53:
A rectangular prism has a length of 5 cm, a width of 4 cm, and a height of 3 cm. What is the volume of the prism?
✅ Answer: 60 cm³ (Volume = l × w × h)
❓ Problem 54:
What do you call a polygon with 8 sides?
✅ Answer: Octagon
❓ Problem 55:
A square has 4 sides of equal length. If each side is 5 cm, what is the perimeter of the square?
✅ Answer: 4 × 5 = 20 cm
Math Word Problems on Time
❓ Problem 56:
If a movie starts at 4:00 PM and ends at 6:30 PM, how long is the movie?
✅ Answer: 2 hours and 30 minutes
❓ Problem 57:
School starts at 8:30 AM and finishes at 3:00 PM. How many hours are you in school?
✅ Answer: 6 hours and 30 minutes
❓ Problem 58:
It’s 7:45 AM now. What time will it be in 1 hour and 15 minutes?
✅ Answer: 9:00 AM
❓ Problem 59:
A train leaves at 10:15 AM and arrives at its destination at 1:45 PM. How long is the train ride?
✅ Answer: 3 hours and 30 minutes
❓ Problem 60:
If a clock shows 9:30, how many minutes are there until 10:00?
✅ Answer: 30 minutes
Measurement and Units
❓ Problem 61:
How many centimeters are there in 1 meter?
✅ Answer: 100 centimeters
❓ Problem 62:
Which is heavier: 1 kilogram of feathers or 1 kilogram of stones?
✅ Answer: They weigh the same
❓ Problem 63:
If a rope is 50 cm long and you cut off 20 cm, how long is the remaining piece?
✅ Answer: 50 cm – 20 cm = 30 cm
❓ Problem 64:
How many milliliters are there in 1 liter?
✅ Answer: 1000 milliliters
❓ Problem 65:
Which unit would you use to measure the length of a book: centimeters or kilometers?
✅ Answer: Centimeters
Money Math Word Problems
❓ Problem 66:
Lucy buys 3 packs of pencils, each containing 4 pencils for $4 per pack. How much does she spend in total?
✅ Solution: 3 × 4 = $12
❓ Problem 67:
You have $50 and buy a toy for $30. If you also want to buy a game for $15, how much money will you have left after both purchases?
✅ Solution: 50 – 30 – 15 = $5
❓ Problem 68:
A sandwich costs $2.50, and a juice costs $1.50. If you pay with a $10 bill, how much change will you receive?
✅ Solution: 10 – (2.50 + 1.50) = $6.00
❓ Problem 69:
If a bag of apples costs $3.00 and you want to buy 4 bags, but you have a coupon that gives you $2 off your total purchase, how much will you pay?
✅ Solution: (4 × 3.00) – 2 = $10.00
❓ Problem 70:
You buy a notebook for $5.75 and a pen for $1.25. If you pay with a $10 bill, how much change will you get?
✅ Solution: 10 – (5.75 + 1.25) = $3.00
Grades 6 to 8 Math Word Problems

Ratios and Proportions
❓ Problem 71:
If the ratio of boys to girls in a class is 3:4 and there are 21 boys, how many girls are there?
✅ Answer: 28 girls
❓ Problem 72:
If 5 kg of apples cost $10, how much would 8 kg cost?
✅ Solution: $16 (Set up the proportion: 5/10 = 8/x)
❓ Problem 73:
In a recipe, the ratio of sugar to flour is 1:4. If you use 2 cups of sugar, how much flour do you need?
✅ Answer: 8 cups of flour
❓ Problem 74:
A map has a scale of 1:50,000. If the distance between two cities on the map is 5 cm, what is the actual distance?
✅ Solution: 2.5 km (Convert cm to km: 5 × 50,000 cm)
❓ Problem 75:
If a car travels 120 km in 2 hours, what is the ratio of distance to time?
✅ Answer: 60 km/h
❓ Problem 76:
The ratio of red marbles to blue marbles in a bag is 3:5. If there are 40 blue marbles, how many red marbles are there?
✅ Answer: 24 red marbles
❓ Problem 77:
A recipe requires a ratio of 2:3 of oil to vinegar. If you use 4 tablespoons of oil, how much vinegar is needed?
✅ Answer: 6 tablespoons of vinegar
❓ Problem 78:
If the proportion of students who play sports is 3 out of 10, how many out of 100 students play sports?
✅ Answer: 30 students
❓ Problem 79:
A car’s fuel efficiency is 15 km per liter. If you have 40 liters, how far can you drive?
✅ Solution: 600 km (Multiply: 15 × 40)
❓ Problem 80:
If a mixture contains 2 parts juice to 5 parts water, what fraction of the mixture is juice?
✅ Answer: 2/7
❓ Problem 81:
A scale model of a house is built at a ratio of 1:100. If the model is 2 meters long, what is the actual length of the house?
✅ Solution: 200 meters (Multiply: 2 × 100)
❓ Problem 82:
In a class of 30 students, the ratio of boys to girls is 2:3. How many boys are in the class?
✅ Answer: 12 boys
❓ Problem 83:
A car’s speed is represented as a ratio of 80 km to 1 hour. If it travels for 3 hours, how far does it go?
✅ Solution: 240 km (Multiply: 80 × 3)
❓ Problem 84:
A fruit basket has apples and oranges in the ratio 4:5. If there are 45 oranges, how many apples are there?
✅ Answer: 36 apples
❓ Problem 85:
If a recipe requires 3 cups of flour for every 2 cups of sugar, how many cups of flour are needed for 8 cups of sugar?
✅ Solution: 12 cups of flour (Set up the proportion)
Math Word Problems on Percentages
❓ Problem 86:
What is 25% of 200?
✅ Answer: 50
❓ Problem 87:
A shirt originally priced at $40 is on sale for 30% off. What is the sale price?
✅ Solution: $28 (Calculate: 40 − (0.30 × 40))
❓ Problem 88:
If 60% of a class of 50 students are girls, how many boys are there?
✅ Answer: 20 boys
❓ Problem 89:
A laptop costs $800 after a 20% discount. What was the original price?
✅ Solution: $1,000 (Use 800 = 0.80 × original price)
❓ Problem 90:
If a population of a town increases by 15% from 1,200, what is the new population?
✅ Solution: 1,380 (Calculate: 1200 + (0.15 × 1200))
❓ Problem 91:
You scored 80 out of 100 on a test. What percentage did you get?
✅ Answer: 80%
❓ Problem 92:
If a $60 item is marked up by 25%, what is the new price?
✅ Solution: $75 (Calculate: 60 + (0.25 × 60))
❓ Problem 93:
A student answered 90 questions correctly out of 120. What percentage did they score?
✅ Solution: 75% (Calculate: 90/120 × 100)
❓ Problem 94:
If you invest $1,000 at an interest rate of 5% per year, how much interest will you earn in one year?
✅ Answer: $50
❓ Problem 95:
In a survey, 80% of participants liked chocolate ice cream. If there were 200 participants, how many liked chocolate?
✅ Answer: 160 participants
Algebraic Expressions
❓ Problem 96:
Simplify the expression: 3x + 4x − 2.
✅ Answer: 7x – 2
❓ Problem 97:
If a = 3, evaluate the expression 2a² + 3a − 5.
✅ Solution: 10 (Calculate: 2(3²) + 3(3) − 5)
❓ Problem 98:
Combine like terms: 5y + 3 − 2y + 7.
✅ Answer: 3y + 10
❓ Problem 99:
If b = 4 and c = 2, what is the value of the expression 2b + 3c − 6?
✅ Solution: 8 (Calculate: 2(4) + 3(2) − 6)
❓ Problem 100:
What is the result of 4(x + 5) − 2x?
✅ Solution: 2x + 20 (Distribute 4 and combine)
❓ Problem 101:
Expand the expression: 2(x + 3) − 4.
✅ Answer: 2x + 2
❓ Problem 102:
If x = 5, find the value of 3x² − 2x + 1.
✅ Solution: 76 (Calculate: 3(5²) − 2(5) + 1)
❓ Problem 103:
Factor the expression: x² + 5x + 6.
✅ Answer: (x + 2)(x + 3)
❓ Problem 104:
What is the value of 7 − 2(3x − 4) when x = 2?
✅ Solution: 9 (Calculate: 7 − 2(3(2) − 4))
❓ Problem 105:
If m = 2 and n = 3, evaluate 4m + 2n − 5.
✅ Solution: 3 (Calculate: 4(2) + 2(3) − 5)
❓ Problem 106:
What is 5(x − 2) + 3(2x + 4) when simplified?
✅ Answer: 11x + 2
❓ Problem 107:
Solve for x in the equation 2x + 3 = 11.
✅ Solution: 4 (Subtract 3 from both sides and divide by 2)
❓ Problem 108:
Combine like terms: 6a − 3 + 4a + 7.
✅ Answer: 10a + 4
❓ Problem 109:
If p = 6, find the value of 2p² − 3p + 4.
✅ Solution: 70 (Calculate: 2(6²) − 3(6) + 4)
❓ Problem 110:
What is the simplified form of 3(2x + 4) − 5(x + 1)?
✅ Answer: x + 7
Math Word Problems on Geometry: Perimeter, Area, and Volume
❓ Problem 111:
Find the perimeter of a rectangle with a length of 8 cm and a width of 5 cm.
✅ Answer: 26 cm
❓ Problem 112:
What is the area of a triangle with a base of 10 cm and a height of 6 cm?
✅ Solution: 30 cm² (Use the formula 1/2 × base × height)
❓ Problem 113:
Calculate the volume of a cube with a side length of 3 cm.
✅ Answer: 27 cm³
❓ Problem 114:
What is the area of a circle with a radius of 7 cm? (Use π ≈ 3.14)
✅ Solution: 153.86 cm² (Use the formula πr²)
❓ Problem 115:
Find the perimeter of a square with a side length of 4 m.
✅ Answer: 16 m
❓ Problem 116:
A rectangular garden has a length of 12 m and a width of 9 m. What is the area?
✅ Solution: 108 m² (Use the formula length × width)
❓ Problem 117:
What is the volume of a cylinder with a radius of 3 cm and a height of 10 cm? (Use π ≈ 3.14)
✅ Solution: 94.2 cm³ (Use the formula πr²h)
❓ Problem 118:
Find the area of a rectangle with a length of 15 cm and a width of 10 cm.
✅ Answer: 150 cm²
❓ Problem 119:
A triangle has sides of lengths 5 cm, 12 cm, and 13 cm. Is it a right triangle?
✅ Answer: Yes
❓ Problem 120:
What is the perimeter of a regular hexagon with a side length of 4 cm?
✅ Answer: 24 cm
Math Word Problems on Speed, Distance, and Time
❓ Problem 121:
If a train travels 120 km in 2 hours, what is its speed?
✅ Answer: 60 km/h
❓ Problem 122:
A cyclist rides at a speed of 15 km/h. How long will it take to travel 45 km?
✅ Solution: 3 hours (Use the formula: Time = Distance/Speed)
❓ Problem 123:
If a car travels at a speed of 80 km/h, how far will it go in 3.5 hours?
✅ Solution: 280 km (Calculate: 80 × 3.5)
❓ Problem 124:
A runner completes a 10 km race in 50 minutes. What was their average speed in km/h?
✅ Solution: 12 km/h (Convert 50 minutes to hours and use the speed formula)
❓ Problem 125:
If a boat moves at a speed of 25 km/h downstream and 15 km/h upstream, what is the speed of the current?
✅ Solution: 5 km/h (Use the formula: Speed downstream – Speed upstream = 2 × speed of the current)
❓ Problem 126:
A car travels 150 km in 2.5 hours. What is its average speed?
✅ Answer: 60 km/h
❓ Problem 127:
If a train leaves a station at 2:00 PM traveling at 90 km/h and arrives at its destination at 4:00 PM, how far did it travel?
✅ Solution: 180 km (Calculate: 90 × 2)
❓ Problem 128:
A person walks 5 km in 1 hour. How long will it take to walk 20 km at the same pace?
✅ Solution: 4 hours (Use the speed formula)
❓ Problem 129:
If a bus travels 300 km at a speed of 75 km/h, how long does the journey take?
✅ Solution: 4 hours (Use the formula: 300/75)
❓ Problem 130:
A runner jogs at a speed of 6 km/h. If they jog for 1.5 hours, how far do they travel?
✅ Answer: 9 km
Integers and Rational Numbers
❓ Problem 131:
What is the sum of -7 and 5?
✅ Answer: -2
❓ Problem 132:
Subtract -3 from 8.
✅ Answer: 11
❓ Problem 133:
Multiply -4 by -6.
✅ Answer: 24
❓ Problem 134:
What is the quotient of -20 divided by 5?
✅ Answer: -4
❓ Problem 135:
If a = -3 and b = 4, what is the value of a + b?
✅ Answer: 1
❓ Problem 136:
Add -15 and 10.
✅ Answer: -5
❓ Problem 137:
What is -12 divided by -3?
✅ Answer: 4
❓ Problem 138:
If you subtract 6 from -2, what do you get?
✅ Answer: -8
❓ Problem 139:
What is the absolute value of -9?
✅ Answer: 9
❓ Problem 140:
Find the product of -7 and 3.
✅ Answer: -21
Data Interpretation Math Word Problems
❓ Problem 141:
A survey shows that 60% of students prefer soccer. If there are 200 students surveyed, how many prefer soccer?
✅ Answer: 120 students
❓ Problem 142:
In a pie chart, 30% represents apples. If the total is 400 fruits, how many apples are there?
✅ Answer: 120 apples
❓ Problem 143:
A bar graph shows that 15 students like math, 10 like science, and 5 like art. What is the total number of students?
✅ Answer: 30 students
❓ Problem 144:
In a class of 40 students, 25% received an A. How many students received an A?
✅ Answer: 10 students
❓ Problem 145:
A line graph shows sales of a product: January – $500, February – $700, March – $600. What is the average sales for these three months?
✅ Answer: $600
❓ Problem 146:
If a survey shows that 40% of people prefer tea over coffee, and 300 people were surveyed, how many prefer tea?
✅ Answer: 120 people
❓ Problem 147:
A group of students scored: 85, 90, 75, 80, and 95. What is the average score?
✅ Solution: 85 (Calculate: (85 + 90 + 75 + 80 + 95)/5)
❓ Problem 148:
In a survey of 50 people, 60% said they exercise regularly. How many people exercise regularly?
✅ Answer: 30 people
❓ Problem 149:
A table shows that 10 students scored A, 15 scored B, and 5 scored C. What is the percentage of students who scored A?
✅ Solution: 20% (Calculate: 10/30 × 100)
❓ Problem 150:
If a class of 30 students had an average score of 70, what is the total score of all students?
✅ Solution: 2,100 (Calculate: 30 × 70)
Before You Go
Before wrapping up your lesson on math word problems, consider these strategies to enhance student understanding and engagement.
- Tailor word problems to reflect real-life scenarios relevant to your students’ interests and experiences.
- Incorporate cross-curricular connections by linking math problems to subjects like science or social studies.
- Encourage peer feedback on problem-solving approaches to foster a collaborative learning environment.
- Experiment with different problem formats to maintain engagement and challenge diverse learning styles.
